The word inequality means a mathematical expression in which the sides are not equal to each other. Basically, an inequality compares any two values and shows that one value is less than, greater than, or equal to the value on the other side of the equation.
Basically, there are five inequality symbols used to represent equations of inequality.
These inequality symbols are: less than (), greater than (>), less than or equal (≤), greater than or equal (≥) and the not equal symbol (≠).
Inequalities are used to compare numbers and determine the range or ranges of values that satisfy the conditions of a given variable.
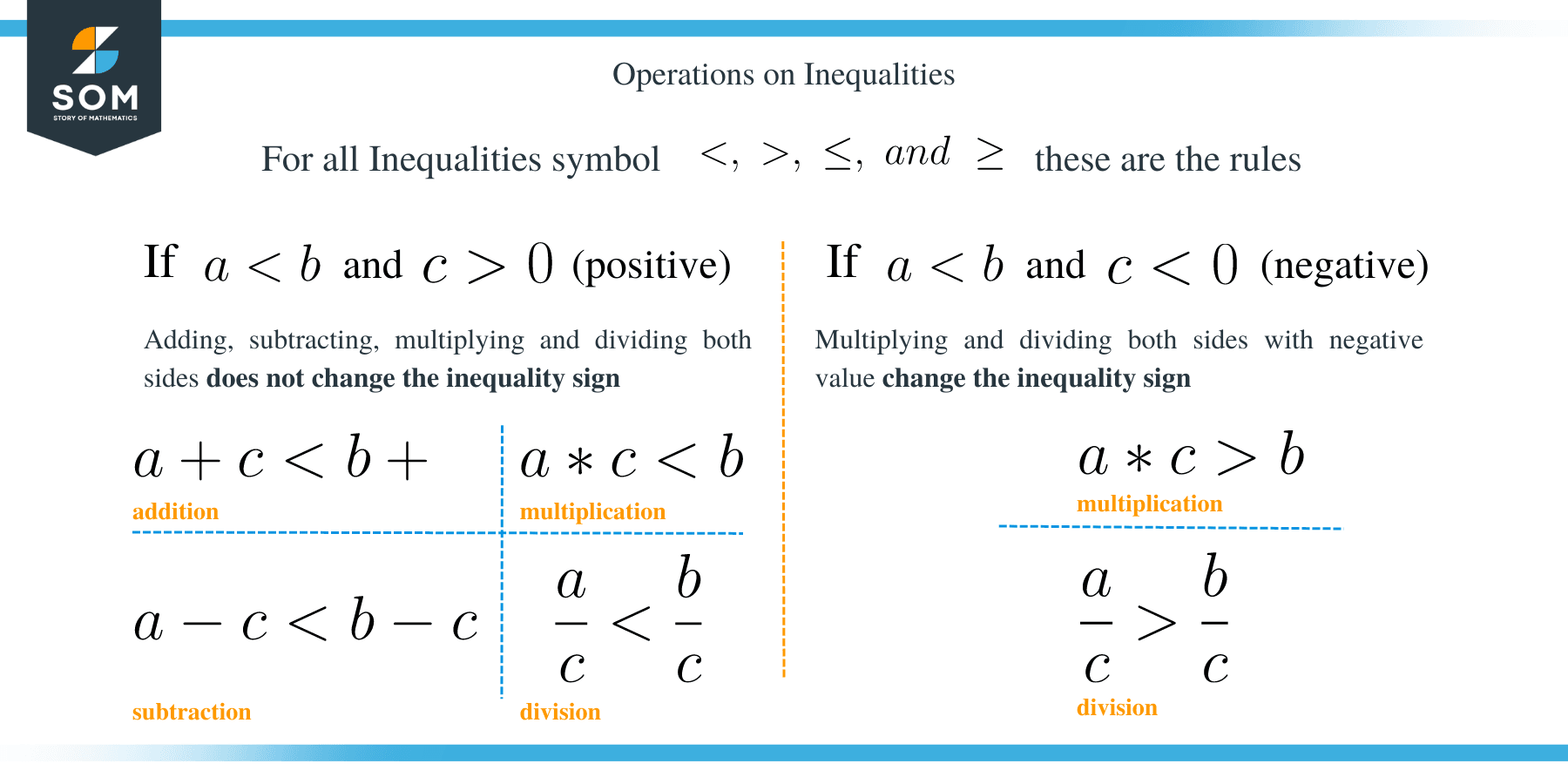
Operations on linear inequalities involve addition, subtraction, multiplication, and division. The general rules for these operations are shown below.

Like linear equations, inequalities can be solved by applying similar rules and steps with a few exceptions. The only difference when solving linear equations is an operation that involves multiplication or division by a negative number. Multiplying or dividing an inequality by a negative number changes the inequality symbol.
Linear inequalities can be solved using the following operations:
Let’s see a few examples below to understand this concept.
Example 1
Solve 3x − 5 ≤ 3 − x.
We start by adding both sides of the inequality by 5
3x – 5 + 5 ≤ 3 + 5 − x
Then add both sides by x.
Finally, divide both sides of the inequality by 4 to get;
Example 2
Calculate the range of values of y, which satisfies the inequality: y − 4 < 2y + 5.
Add both sides of the inequality by 4.
Subtract both sides by 2y.
Let’s see a few examples below to understand this concept.
Example 3
Isolate the variable x by subtracting 8 from both sides of the inequality.
x + 8 – 8 > 5 – 8 => x > −3
Example 4
Solve 5x + 10 > 3x + 24.
Subtract 10 from both sides of the inequality.
5x + 10 – 10 > 3x + 24 – 10
Now we subtract both sides of the inequality by 3x.
5x – 3x > 3x – 3x + 14
Let’s see a few examples below to understand this concept.
Example 5
Multiply both sides of an inequality by the denominator of the fraction
Example 6
Multiply both sides of an inequality by 4.
Multiply both sides of the inequality by -1 and reverse the direction of the inequality symbol.
Let’s see a few examples below to understand this concept.
Example 7
Solve the inequality: 8x − 2 > 0.
First of all, add both sides of the inequality by 2
Now, solve by dividing both sides of the inequality by 8 to get;
Example 8
Solve the following inequality:
Divide both sides of the inequality by -5 and change the direction of the inequality symbol
Let’s see a few examples below to understand this concept.
Example 9
Solve: 2 (x – 4) ≥ 3x – 5
Apply the distributive property to remove the parentheses.
Add both sides by 8.
⟹ 2x – 8 + 8 ≥ 3x – 5 + 8
Subtract both sides by 3.
⟹ 2x – 3x ≥ 3x + 3 – 3x
Example 10
A student scored 60 marks in the first test and 45 marks in the second test of the terminal examination. How many minimum marks should the student score in the third test get a mean of least 62 marks?
Let the marks scored in the third test be x marks.
(60 + 45 + x)/3 ≥ 62
105 + x ≥ 196
x ≥ 93
Therefore, the student must score 93 marks to maintain a mean of at least 62 marks.
Example 11
Justin requires at least $500 to hold his birthday party. If already he has saved $ 150 and 7 months are left to this date. What is the minimum amount he must save monthly?
Let the minimum amount saved monthly = x
150 – 150 + 7x ≥ 500 – 150
Therefore, Justin should save $50 or more
Example 12
Find two consecutive odd numbers which are greater than 10 and have the sum of less than 40.
Let the smaller odd number = x
Therefore, the next number will be x + 2
x > 10 ………. greater than 10
Solve the equations.
Combine the two expressions.
Therefore, the consecutive odd numbers are 11 and 13, 13 and 15, 15 and 17, 17 and 19.
The best tool to represent and visualize numbers is the number line. A number line is defined as a straight horizontal line with numbers placed along at equal segments or intervals. A number line has a neutral point at the middle, known as the origin. On the right side of the origin on the number line are positive numbers, while the left side of the origin is negative numbers.
Linear equations can also be solved by a graphical method using a number line. For example, to plot x > 1, on a number line, you circle the number 1 on the number line and draw a line going from the circle in the direction of the numbers that satisfies the inequality statement.
Example 13
![]()
If the inequality symbol is greater than or equal to or less than or equal to sign (≥ or ≤), draw the circle over the numerical number and fill or shade the circle. Finally, draw a line going from the shaded circle in the numbers’ direction that satisfies the inequality equation.
Example 14
![]()
The same procedure is used to solve equations involving intervals.
Example 15
![]()
Example 16
![]()
Example 17